| ENG RUS | Timus Online Judge |
1764. TranssibTime limit: 0.5 second Memory limit: 64 MB The route of the Moscow–Vladivostok “Rossiya” passenger train is considered to be the main route of the Trans-Siberian Railway. Its itinerary includes
Nizhny Novgorod, Kirov, Perm, and Yekaterinburg. The train goes from Moscow to
Yekaterinburg in 25 h 41 min. The “Ural” train follows the southern line of
the Trans-Siberian Railway through Kazan and completes the journey in 25 h 25
min. It is impossible to go by train from Moscow to Yekaterinburg in a shorter
time. 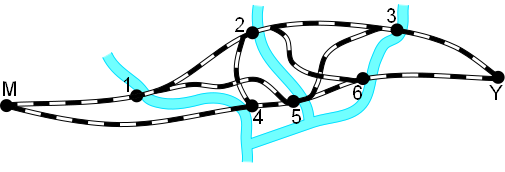 This is the scheme of the railroads between Moscow (M) and
Yekaterinburg (Y). It is seen that the routes of the trains
“Rossiya” (the upper line in the scheme) and “Ural” (the lower line) are
crossed by major rivers: Volga, Vyatka, and Kama. The former train crosses them
at Nizhny Novgorod (1), Kotelnich (2), and Perm (3), respectively. The latter
train crosses the rivers in 35 km west of Kazan (4), in Vyatskie Polyany (5),
and in Sarapul (6). The scheme also shows direct lines connecting some of these
cities. In addition to passenger trains, there are also goods trains following these
railroads. They can take one of the four routes:
Minister of Railway Transport wants to organize the goods train service in such
a way that the freight flow from Moscow to Yekaterinburg be as much as
possible. He knows that the bridges across the rivers shown in the scheme are
the “bottlenecks” for the trains. For each bridge, the carrying capacity is
known, i.e., the amount of freight that can be taken through the bridge in a
day. Help the minister solve the problem. InputThe only input line contains the carrying capacities of the bridges in Nizhny
Novgorod, in Kotelnich, in Perm, near Kazan, in Vyatskie Polyany, and in
Sarapul. These are integers in the range from 1 to 109. OutputFind the daily amount of freight sent from Moscow along each of the
routes specified above so that the total freight flow from Moscow to
Yekaterinburg be maximal. Output these four numbers accurate to 10−3,
separating them with a space. If the problem has several solutions, output any
of them. Sample
Problem Author: Alexander Ipatov Problem Source: The 14th Urals Collegiate Programing Championship, April 10, 2010 Tags: none |
